Molarity
The content that follows is the substance of lecture 10. In this lecture we cover Molarity, Units of Concentration and the Dilution Process.
Solution Concentration is an important underlying concept that you should know well before we start the next few lectures on Solutions. In other words you can't understand how to convert and use information regarding solutions unless we first discuss the units in which the concentration of said solutions will be expressed.
There are Five main ways we describe the concentration of solutions: 1) Molarity; 2) Molality; 3) Weight Percent; 4) Mole Fraction; and 5) Parts Per Million or Billion. You should know the meaning of each of these terms and more importantly how to convert from one to the other. I have added a conversion calculator at the bottom of this page to help you check your homework etc. But don't become too reliant on it since it will not be available during exams.
Molarity: The molarity of a solution is calculated by taking the moles of solute and dividing by the liters of solution. Molarity is designated by a capital "M".
Molarity = Moles Solute / Liter of Solution
Molality: The molality of a solution is calculated by taking the moles of solute and dividing by the kilograms of solvent. Molality is designated by a lower case "m". We often express concentrations in molality when we publish because unlike molarity, molality is not temperature dependent. This independence makes it easier for scientists around the world to reproduce the work. There is a simple method for converting molarity to molality:
Here is a simple example:
Find the molality of 2.5 M H2SO4. This solution has a density of 1.54 g/mL.
Step 1. Make an assumption. Assume you have 1 L of solution. This is a very important step and the amount of solution is not given but you need to have a specific quantity to do the calculations and one liter is the best assumption for this problem.
Step 2. Find the total mass of the solution. Multiply 1 L X the density (1.54 g/mL) X 1000 mL/L. This gives 1540 grams of solution.
Step 3. Calculate the grams of the solute. 2.5M means 2.5 moles of sulfuric acid per one liter of solution. Convert 2.5 moles to grams. The molar mass of sulfuric acid is 98.09 g/mol. 2.5 moles X 98.09 g/mol = 245.225 grams of sulfuric acid.
Step 4. Calculate the grams of the solvent. 1540 grams of solution - 245.225 grams of solute = 1294.775grams or 1.294775 kg solvent
Step 5. Calculate the molality. 2.5 moles solute / 1.294775 kg solvent = 1.9 molal H2SO4
Weight Percent(or Mass Percent): The weight percent of a solution is calculated by taking the mass of a single solute and dividing it by the total mass of the solution. This can be in grams or kilograms as long as the units of both are expressed in the same manner. The ratio is then multiplied by 100%. The weight percent is designated by Wt% and sometimes w/w%.

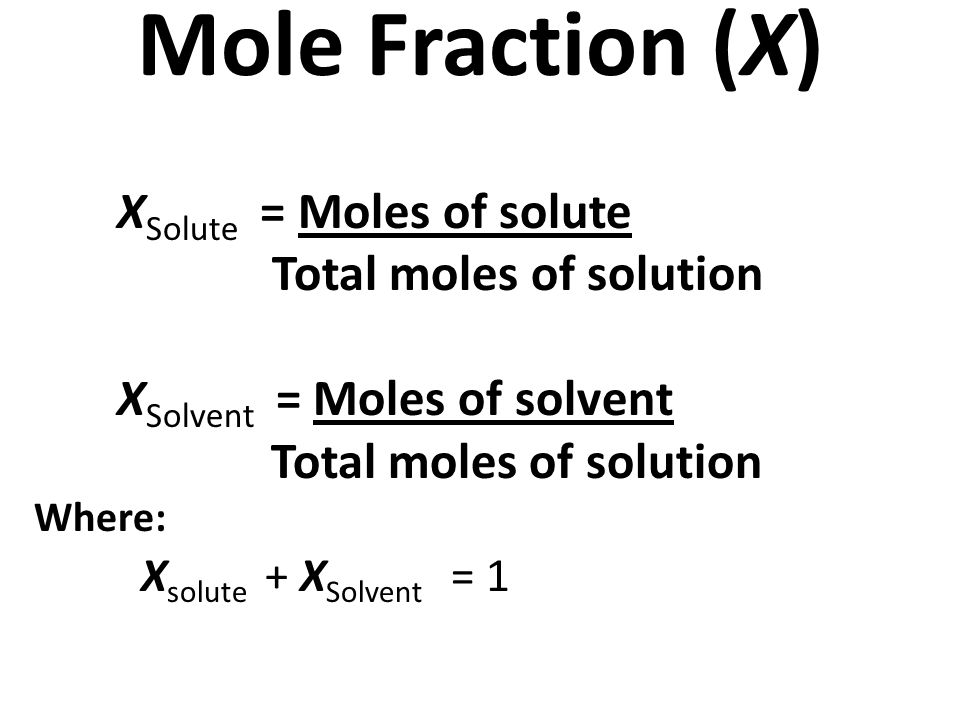
Mole Fraction: The mole fraction of a single solute in a solution is simply the number of moles of that solute divided by the total moles of all the solutes/solvents. The mole fraction of solute i is written as Xi.
Parts Per Million(PPM) and Parts per Billion (PPB): "Parts per" is a convenient notation used for low and very low concentrations. Generally speaking it is very similar to weight percentage - 1% w/w means 1 gram of substance per every 100 g of sample and it is (although very rarely) named pph - parts per hundred. Other abbreviations stand for:
| ppm | parts per milion (106) |
| ppb | parts per bilion (109) |
| ppt | parts per trillion (1012) |
| ppq | parts per quadrilion (1015) |
ppq is more a theoretical construct than a useful measurement, chances are you will never see it in use. Parts per million also can be expressed as milligrams per liter (mg/L).
Serial Dilution:
Serial dilution is a process by which a series of solutions can be made which conserves the amount of chemical needed. The process uses each successively created solution as the stock solution for the next. The calculation is very simple. The Equation:
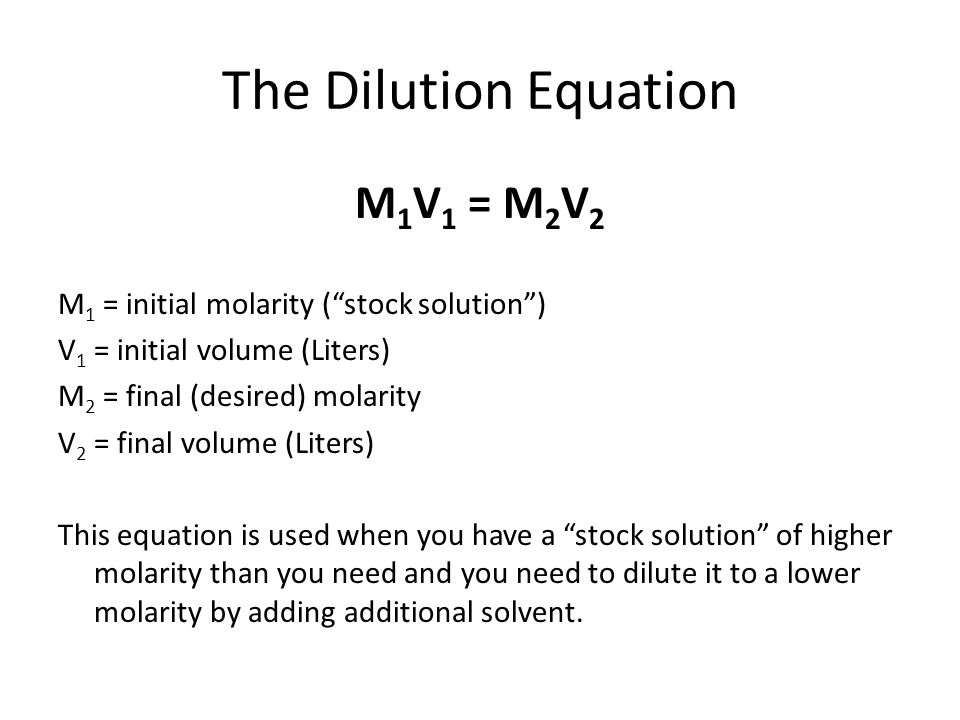
The equation allows you to calculate how much of the stock solution contains the right amount of moles for the more dilute solution. Note: You can only go from higher to lower concentration. You cannot "concentrate" a solution from lower to higher concentration. The following video runs through the calculations in more detail.
Practice Problems: